Login form
SAT - Grid-ins - Test 4
in Grid-ins
Solve the problem and enter the calculated result to the text box.
1. A typist can type 45 words per minute. He increases his speed by 20 per cent. How many words can he now type per hour ?
2. If 2y - x = 8 , and 3x - y = 1, what is the value of x ?
3. The sum of four consecutive integers is 410. What is the value of the least of these integers?
4. On a map showing only four countries, A, B, C and D, A shares a border with B and C. Country D shares a border with B and C. But countries B and C and countries A and D do not share borders. If the map requires different colours for countries with common borders, what is the minimum number of colours required to complete the map?
5. A square has sides s and diagonal d. If 2s² + d² = 100, what is the value of s?
6. Different four-letter passwords can be constructed using the letters A, B, C and D only once. How many such passwords exist if either C or B must be in second position?
7. A book distributor sends out standardized packages weighing 1, 1.5 or 2 kilograms. If during one week 40 per cent of the packages weigh 1 kg, 50 per cent weigh 1.5 kg and 10 per cent weigh 2 kg, what is the average weight in kilograms of the parcels that week?
8. If two lines intersect at apoint to form four angles, and one angle is twice as large as its adjacent (neighboring) angle, what is the degree measure of the smallest angle?
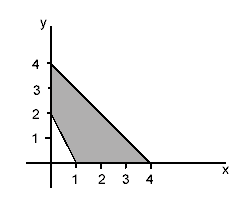
9. What is the area of the shaded region?